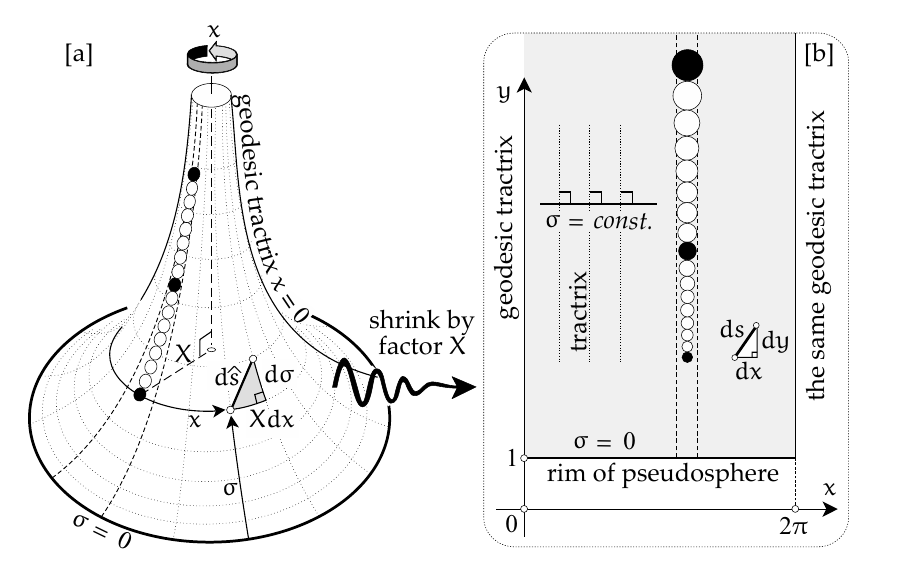
Pseudosphere
It is the surface in $\mathbb{R}^3$ that appears when you rotate the tractrix
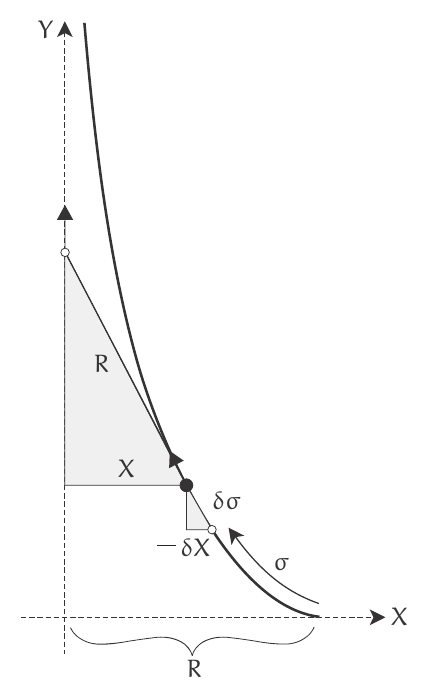
It can be shown that if $\sigma$ is the arc-length parametrization of the tractrix then
$$ X=Re^{-\sigma/R} $$The pseudosphere has $K=-1/R^2$, where $R$ is its "base radius".
It is a model for the hyperbolic plane but is not "really good" because it has a boundary and it is "wraped". So usually is preferred another model, obtained has a conformal chart of the pseudosphere, the Poincare half plane (@needham2021visual page 54-55).
________________________________________
________________________________________
________________________________________
Author of the notes: Antonio J. Pan-Collantes
INDEX: